Jul 06
Interleaved Learning
Tanmay Vora (@tnvora) has two very nice sketchnotes and associated articles on learning.
Tanmay Vora’s associated articles are here:
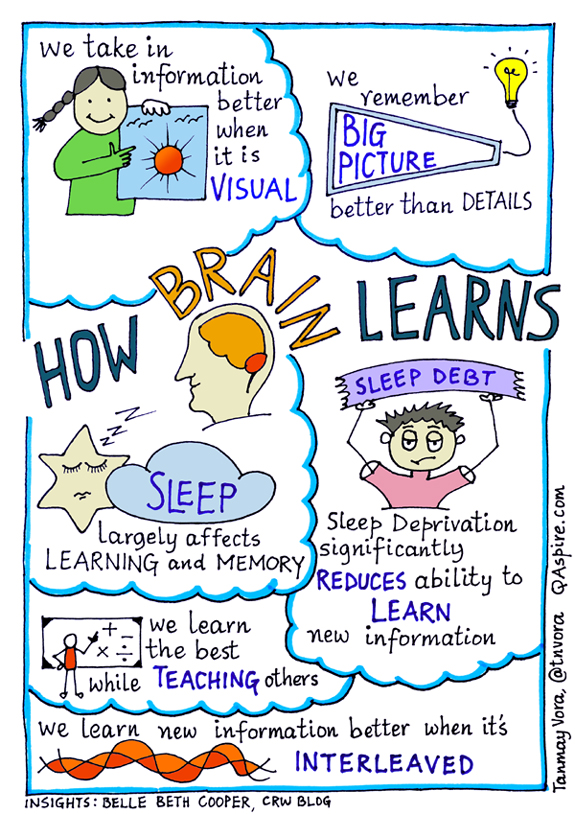
- How Our Brain Learns
- Which is adapted from the article (by Belle Beth Cooper) 6 important things you should know about how your brain learns (#6 is Interleaved Learning)
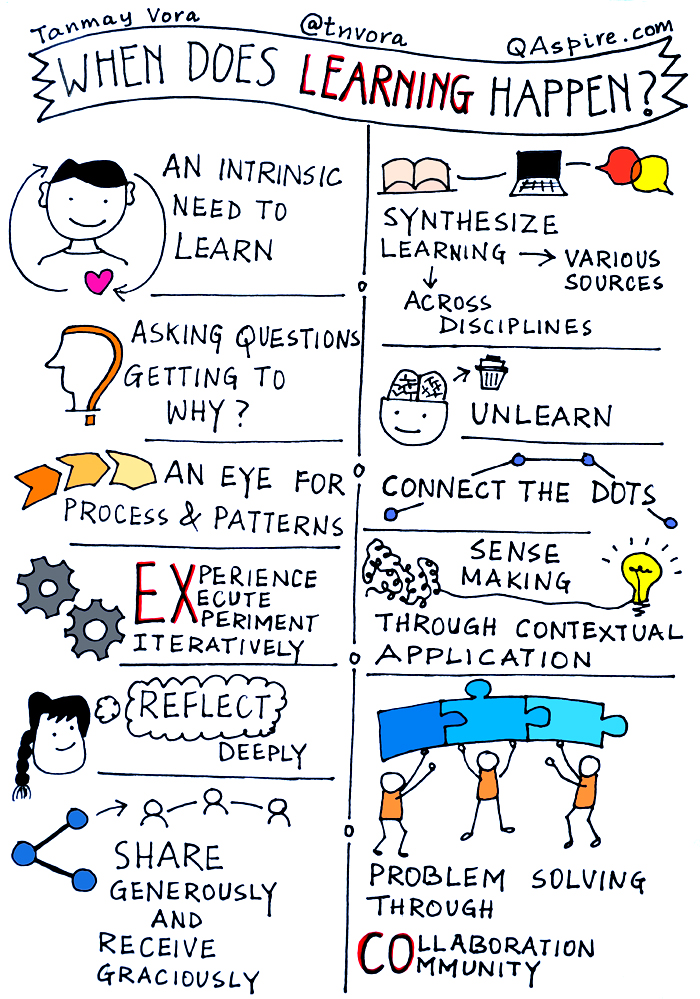
- When Does Real Learning Happen?
I was especially struck by the bottom of the sketchnote that says We learn new information better when it’s INTERLEAVED.
This may be a new term to many and it is a fairly new idea in terms of educational research.
Scientific American had a nice article in August 2015 on this (http://www.scientificamerican.com/article/the-interleaving-effect-mixing-it-up-boosts-learning/). The article talks about math quite a bit. There are numerous links to research and other articles. It contains many good points, including two reasons why interleaving strengthens memory associations.
Put simply, interleaved learning is when students practice in an ABCABCABC pattern rather than (in blocks) AAABBBCCC. As a teacher, I have long been an advocate of mixed practice. Interleaved learning is related to, but not equivalent to, Distributed Practice.
Give interleaved learning a try.
Nov 03
OK Everybody get your shoes on….
I like to use the following story of a parent interacting with their 3-year-old to make a point.
Parent: “OK Everybody get your shoes on.”
3-year-old: “Why?”
Parent: “Because we need to get in the van.”
3-year-old: “Why?”
Parent: “Because we need to go to WalMart.”
3-year-old: “Why?”
Parent: “Because we need to get some groceries.”
3-year-old: “Why?”
Parent: “Because we don’t have any food and if we don’t buy some groceries, we will not eat lunch.”
3-year-old: “Oh, OK, I’ll get my shoes on.”
The principle I’m illustrating is the technique the 3-year-old is already using. He wants to know how the world works. As humans, we an an innate desire for things to make sense. We an an innate desire to know why. 3-year-olds do this instinctively. Unfortunately, sometimes people loose this desire to ask why.
Notice how the technique is used. The child did not just ask why once. As the parent and child play this game, the parent knows that this will only end when the answer is something that is inherently true, foundational (so the parent is trying to work to an answer that is so obviously true that the why-asking can end).
In mathematics (and school in general) we should always ask Why — and we should ask, in response to each answer, why again. In this way we often work our way to foundational truths – which are very important truths. Furthermore, connecting foundational truths to today’s problem adds powerful connections in the brain.
This technique of asking why repeatedly until a foundational truth, or underlying cause or principle is found has been studied and advocated by Sakichi Toyoda and was used within the Toyota Motor Corporation during the evolution of its manufacturing methodologies. See https://en.m.wikipedia.org/wiki/5_Whys
After you solve a math problem, in the Look Back and Extend phase (among other things) ask WHY.
Oct 04
Top 20 Principles from Psychology for PreK–12 Teaching and Learning
Top 20 Principles from Psychology for PreK–12 Teaching and Learning
Feb 16
Research Terms Defined
This is a nice infographic on terminology. Validity, positivism, etc.
There is a good list of sources at the bottom of the infographic.
Feb 16
My Original ‘Plethora’ Page
A couple years ago, I made a fairly extensive page on research, titled, Math Education Research and a Plethora of Committee and Government Reports.
With this website coming on board, I probably won’t be editing that page much (we’ll see). I will probably make this site my main workhorse when it comes to Math Education Research and the like.
Feb 16
Under construction
This site is (new as of 2/15/15) under construction. Yep, it needs to be organized.
Feb 16
Why Johnny Can’t Add Without a Calculator
“Technology is doing to math education what industrial agriculture did to food: making it efficient, monotonous, and low-quality.”
This is pretty (OK, quite) negative on technology. I don’t think it claims (or the reader should conclude) that all technology is bad.
It offers some very realistic concerns. The article is fairly extensive.